In Fig. 6.62, two chords AB and CD of a circle intersect each other at the point P(when produced) outside the circle. Prove that

(i) Δ PAC ~ Δ PDB

(ii) PA . PB = PC . PD

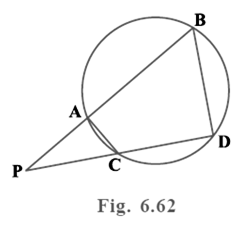
(i) In triangle PAC and PDB

∠PAC + ∠CAB = 180^o (Linear pair)

∠CAB + ∠BDC = 180^O (Opposite angles of a cyclic quadrilateral are supplementary)

Hence,

∠PAC = ∠PDB

Similarly,

∠PCA = ∠PBD

Hence,

Δ PAC ~ Δ PDB

(ii) Since the two triangles are similar, so

Or,

PA * PB = PC * PD

Hence, proved