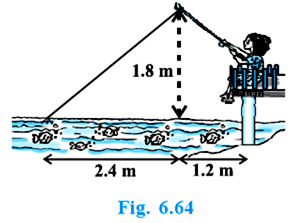
Nazima is fly fishing in a stream. The tip ofher fishing rod is 1.8 m above the surfaceof the water and the fly at the end of thestring rests on the water 3.6 m away and2.4 m from a point directly under the tip ofthe rod. Assuming that her string(from the tip of her rod to the fly) is taut,how much string does she have out(see Fig. 6.64)? If she pulls in the string atthe rate of 5 cm per second, what will bethe horizontal distance of the fly from herafter 12 seconds?

As per the question:

AD = 1.8 m

BD = 2.4 m

CD = 1.2 m

Retracting speed of string = 5 cm per second

In triangle ABD, length of string i.e. AB can be calculated as follows

AB² = AD² + BD²

= (1.8)² + (2.4)²

= 3.24 + 5.76

= 9

Or, AB = 3

Let us assume that the string reaches at point M after 12 seconds

Length of refracted string in 12 inches = 5 * 12 = 60 cm

Remaining length = 3 – 0.6 = 2.4 m

In triangle AMD, we can find MD by using Pythagoras theorem,

MD² = AM² – AD²

= 2.4² – 1.8²

= 5.76 – 3.24

= 2.52

Or, MD = 1.58

Hence,

Horizontal distance between the girl and the fly = CD + MD

= 1.2 + 1.58

= 2.78 m