Show that where a,b,c are in A.P.

Let

Recall that the value of a determinant remains same if we apply the operation R_i→ R_i + kR_j or C_i→ C_i + kC_j.

Applying R₁→ R₁ + R₃, we get

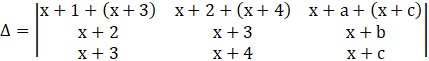
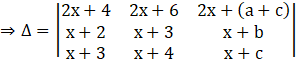
Given that a, b and c are in an A.P. Using the definition of an arithmetic progression, we have

b – a = c – b

⇒ b + b = c + a

⇒ 2b = c + a

∴ a + c = 2b

By substituting this in the above equation to find Δ, we get

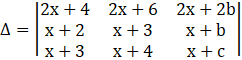
Taking 2 common from R₁, we get

Applying R₁→ R₁ – R₂, we get

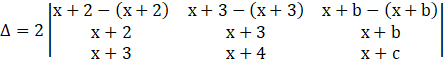
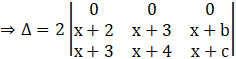
∴ Δ = 0

Thus, when a, b and c are in A.P.