Solve the following determinant equations:

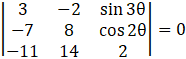
Let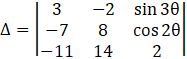

We need to find the roots of Δ = 0.

Recall that the value of a determinant remains same if we apply the operation R_i→ R_i + kR_j or C_i→ C_i + kC_j.

Applying C₁→ C₁ + C₂, we get

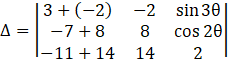
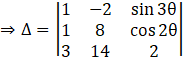
Applying R₂→ R₂ – R₁, we get

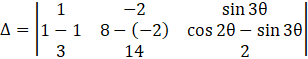
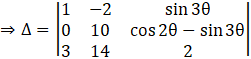
Applying R₃→ R₃ – 3R₁, we get

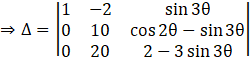
Expanding the determinant along C₁, we have

Δ = (1)[(10)(2 – 3sin(3θ)) – (20)(cos(2θ) – sin(3θ))]

⇒ Δ = [20 – 30sin(3θ) – 20cos(2θ) + 20sin(3θ)]

⇒ Δ = 20 – 10sin(3θ) – 20cos(2θ)

From trigonometry, we have sin(3θ) = 3sinθ – 4sin³θ and cos(2θ) = 1 – 2sin²θ.

⇒ Δ = 20 – 10(3sinθ – 4sin³θ) – 20(1 – 2sin²θ)

⇒ Δ = 20 – 30sinθ + 40sin³θ – 20 + 40sin²θ

⇒ Δ = –30sinθ + 40sin²θ + 40sin³θ

∴ Δ = 10(sinθ)(–3 + 4sinθ + 4sin²θ)

The given equation is Δ = 0.

⇒ 10(sinθ)(–3 + 4sinθ + 4sin²θ) = 0

⇒ (sinθ)(–3 + 4sinθ + 4sin²θ) = 0

Case – I:

sin θ = 0 ⇒ θ = kπ, where k ϵ Z

Case – II:

–3 + 4sinθ + 4sin²θ = 0

⇒ 4sin²θ + 4sinθ – 3 = 0

⇒ 4sin²θ + 6sinθ – 2sinθ – 3 = 0

⇒ 2sinθ(2sinθ + 3) – 1(2sinθ + 3) = 0

⇒ (2sinθ – 1)(2sinθ + 3) = 0

⇒ 2sinθ – 1 = 0 or 2sinθ + 3 = 0

⇒ 2sinθ = 1 or 2sinθ = –3

or

However, as –1 ≤ sin θ ≤ 1.

, where k ϵ Z

Thus, kπ and for all integral values of k are the roots of the given determinant equation.