Using determinants, find the area of a triangle whose vertices are (1, 4), (2, 3) and (– 5, – 3). Are the given points collinear?

Given: – Vertices are (1, 4), (2, 3) and (– 5, – 3)

Tip: – If vertices of a triangle are (x₁,y₁), (x₂,y₂) and (x₃,y₃), then the area of the triangle is given by:

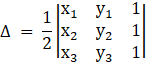
Now,

Substituting given value in above formula

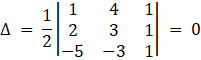
Expanding along R₁

⇒

⇒

⇒

Since area can’t be negative

⇒

Tip: – For Three points to be collinear, the area of the triangle formed by these points will be zero

Now, as the area is not zero

Therefore, Points (1, 4), (2, 3) and (– 5, – 3) are not collinear.