Find the real values of λ for which the followings system of linear equations has non – trivial solutions. Also, find the non – trivial solutions
2λx – 2y + 3z = 0
X + λy + 2z = 0
2x + λz = 0
Given Equations:
2λx – 2y + 3z = 0
x + λy + 2z = 0
2x + λz = 0
For trivial solution D = 0

![]()
= 2λ (λ×λ – 0×2) + 2(1×λ – 2×2) + 3(1×0 – 2×λ)
= 2λ (λ2 – 0) + 2(λ – 4) + 3(0 – 2λ)
= 2λ3 + 2λ – 8 – 6λ
= 2λ3 + 4λ – 8
Now D = 0
2λ3 – 4λ – 8 = 0
2λ3 – 4λ = 8
λ(λ2 – 2) = 4
Hence λ = 2
Now let z = k
⇒ 4x – 2y = – 3k
And x + 2y = – 2k
Now using the cramer’s rule
![]()
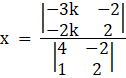
![]()
x = – k
similarly,
![]()
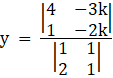
![]()
![]()
![]()
4