Find all the points of discontinuity of f defined by
f(x) = |x| – |x + 1|.
Basic Idea:
A real function f is said to be continuous at x = c, where c is any point in the domain of f if :
![]() where h is a very small ‘+ve’ no.
where h is a very small ‘+ve’ no.
i.e. left hand limit as x → c (LHL) = right hand limit as x → c (RHL) = value of function at x = c.
This is very precise, using our fundamental idea of the limit from class 11 we can summarise it as a function is continuous at x = c if :
![]()
NOTE: ![]()
Here we have,
f(x) = |x| – |x + 1|
f(x) rewritten using idea of mod function:
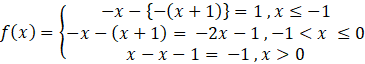 …….equation 1
…….equation 1
Clearly for x < –1 , f(x) = constant and also for x > 1 f(x) is constant
∴ in these regions f(x) is everywhere continuous.
For –1 < x < 0, plot of graph is a straight line as in this region f(x) is given by linear polynomial
∴ it is also continuous here.
∵ function is changing its expression at x = –1 and x = 0, so we need to check continuities at these points.
At x = –1 :
f(–1) = 1 [using equation 1]
LHL = ![]() [using equation 1]
[using equation 1]
RHL = ![]()
[using equation 1]
Clearly,
LHL = RHL = f(–1)
∴ it is continuous at x = –1
At x = 0 :
f(0) = –2*0–1 = –1 [using equation 1]
LHL = ![]()
[using equation 1]
RHL = ![]()
[using equation 1]
Clearly,
LHL = RHL = f(0)
∴ it is continuous at x = 0
Hence,
f(x) is continuous everywhere in its domain.