A TV tower stands vertically on a bank of a canal. From a point on the other bank directly opposite the tower, the angle of elevation of the top of the tower is 60°. From another point 20 m away from this point on the line joining this point to the foot of the tower, the angle of elevation of the top of the tower is 30° (see Fig. 9.12). Find the height of the tower and the width of the canal
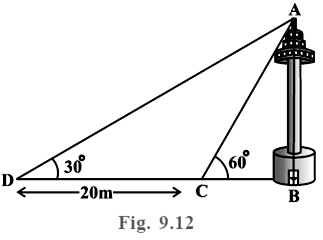
According to this figure:
CD = 20 m,
∠ACB = 60°,
∠ ADB = 30°,
AB = ? and BD = ?
In Δ ABC,
![]()
![]()
![]()
![]()
In Δ ABD,
![]()
![]()
![]()
𝐹rom (i) and (ii),
![]()
![]()
![]()
Using the value of BC in (i) we get;
AB = 10√3 m which is the height of the tower
Width of canal = 10 m
11