Using differentials, find the approximate values of the following:

Question

Philoid · Accepted Answer

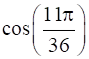
Let us assume that f(x) = cos x

Let so that

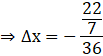
On differentiating f(x) with respect to x, we get

We know

When, we have.

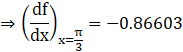
Recall that if y = f(x) and Δx is a small increment in x, then the corresponding increment in y, Δy = f(x + Δx) – f(x), is approximately given as

Here, and

⇒ Δf = (–0.86603)(–0.0873)

∴ Δf = 0.07560442

Now, we have

Thus,