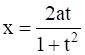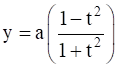Show that the point (x, y) given by and lies on a circle for all real values of t such that - 1 ≤ t ≤ 1, where a is any given real number.

Question

Philoid · Accepted Answer

Given:

⇒

We need to prove that the point (x, y) lies on a circle for real values of t such that - 1≤t≤1, where a is any given real number.

Consider x² + y²,

⇒

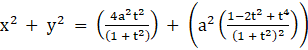
⇒ x² + y² = a²

The point (x,y) lies on a circle.

∴Thus proved.