find the equation of the ellipse in the following cases:

eccentricity 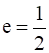and major axis = 12

Given that we need to find the equation of the ellipse whose eccentricity is and the major axis is 12.

Let us assume the equation of the ellipse as (a²>b²).

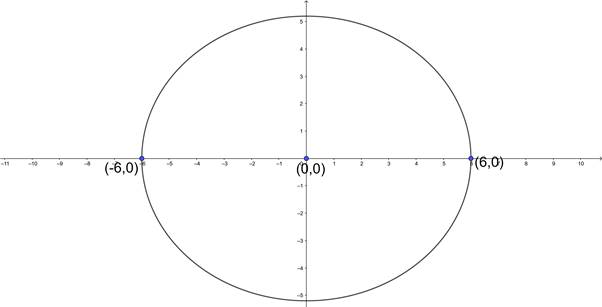
We know that eccentricity(e) =

⇒

⇒

⇒

⇒

We know that length of ,ajor axis is 2a.

⇒ 2a = 12

⇒ a = 6

⇒ a² = 36

⇒

⇒ b² = 27

The equation of the ellipse is

⇒

⇒

⇒ 3x² + 4y² = 108

∴ The equation of the ellipse is 3x² + 4y² = 108.