A particle is moving in a straight line such that its distance s at any time t is given by 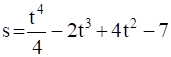. Find when its velocity is maximum and acceleration minimum.

Question

Philoid · Accepted Answer

Given:

The distance covered by a particle at time ‘t’ is given by,

⇒

We know that velocity of a particle is given by and acceleration of a particle is given by .

⇒

We need velocity to be maximum,

We know that for maxima and minima,

⇒

Differentiating ‘v’ again,

⇒

At

⇒

⇒ >0(Minima)

At

⇒

⇒ <0(Maxima)

We get the velocity maximum at

Now, we find the acceleration:

⇒

⇒ a = 3t² - 12t + 8

We need acceleration to be minimum,

We know that for maxima and minima,

⇒

⇒ t = 2

Differentiating ‘a’ again,

⇒

At t = 2

⇒ >0(Minima)

We get minimum for t = 2 ,

∴ we get maximum velocity at and minimum acceleration at t = 2