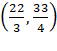Find the values of the parameter a so that the point (a, 2) is an interior point of the triangle formed by the lines x + y – 4 = 0, 3x – 7y – 8 = 0 and 4x – y – 31 = 0.

Question

Philoid · Accepted Answer

Given:

x + y – 4 = 0, 3x – 7y – 8 = 0 and 4x – y – 31 = 0 forming a triangle and point (a, 2)is an interior point of the triangle

To find:

Value of a

Explanation:

Let ABC be the triangle of sides AB, BC and CA whose equations are x + y − 4 = 0, 3x − 7y − 8 = 0 and 4x − y − 31 = 0, respectively.

On solving them, we get A (7, - 3), B and C 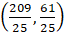as the coordinates of the vertices.
Let P (a, 2) be the given point.

Diagram:

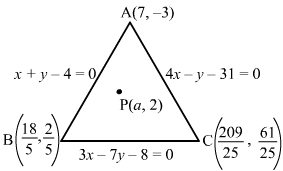
It is given that point P (a, 2) lies inside the triangle. So, we have the following:

(i) A and P must lie on the same side of BC.

(ii) B and P must lie on the same side of AC.

(iii) C and P must lie on the same side of AB.

Thus, if A and P lie on the same side of BC, then

21 + 21 – 8 – 3a – 14 – 8 > 0

⇒ a > … (1)

If B and P lie on the same side of AC, then

⇒ a < … (2)

If C and P lie on the same side of AB, then

⇒

⇒ a > 2 … (3)

From (1), (2) and (3), we get:

A ∈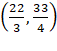

Hence, A ∈