Draw a right triangle in which the sides (other than hypotenuse) are of lengths 5 cm and 4 cm. Then construct another triangle whose sides are  times the corresponding sides of the given triangle.
times the corresponding sides of the given triangle.
Steps of construction:
1. BC=4cm is drawn.

2. At B, a ray of 5 cm making an angle of 90° with BC is drawn at A. Join AC.
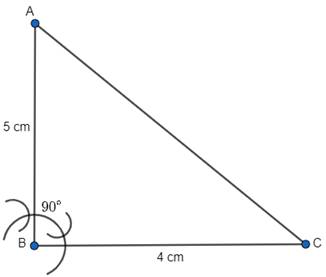
3. A ray BX is drawn making an acute angle with BC opposite to vertex A. Five points B1 , B2 , B3, B4, and B5 at equal distance is marked on BX.
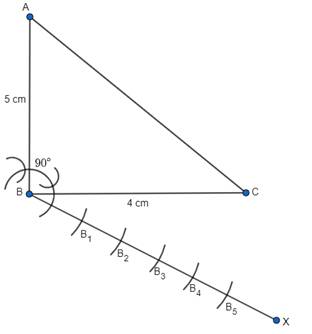
4. B3C is joined B5C1 is made parallel to B3C.
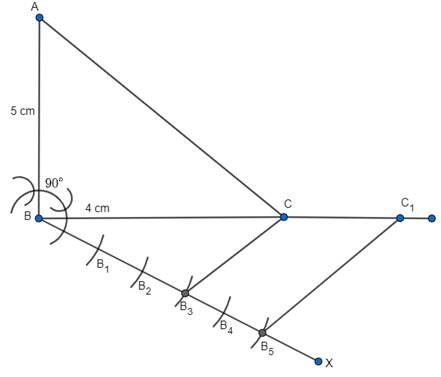
5. Now A1C1 is made parallel to AC.
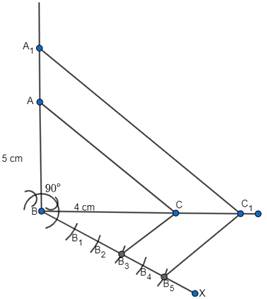
Triangle A1BC1 is the required triangle.
Justification:
Since the scale factor is ![]() ,
,
We need to prove,
![]()
By construction,
![]() … (1)
… (1)
Also, A1C1 is parallel to AC.
So, this will make same angle with BC.
∴ ∠A1C1B = ∠ACB …. (2)
Now,
In ΔA1BC1 and ΔABC
∠ B = ∠ B (common)
∠A1C1B = ∠ACB (from 2)
ΔA1BC1∼ ΔABC
Since corresponding sides of similar triangles are in same ratio.
![]()
From (1)
![]()
Hence construction is justified.