Find values of a and b if A = B, where

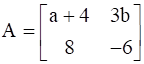and

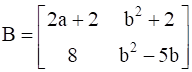
We have the matrices A and B, where

We need to find the values of a and b.

We know that, if

Then,

a₁₁ = b₁₁

a₁₂ = b₁₂

a₂₁ = b₂₁

a₂₂ = b₂₂

Also, A = B.

This means,

a + 4 = 2a + 2 …(i)

3b = b² + 2 …(ii)

8 = 8

-6 = b² – 5b …(iii)

From equation (i), we can find the value of a.

a + 4 = 2a + 2

⇒ 2a – a = 4 – 2

⇒ a = 2

From equation (ii), we can find the value of b².

3b = b² + 2

⇒ b²= 3b – 2

Substitute the value of b² in equation (iii), we get

-6 = b² – 5b

⇒ -6 = (3b – 2) – 5b

⇒ -6 = 3b – 2 – 5b

⇒ -6 = 3b – 5b – 2

⇒ -6 = -2b – 2

⇒ 2b = 6 – 2

⇒ 2b = 4

⇒ b = 2

Thus, a = 2 and b = 2.