If 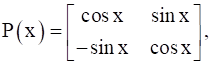then show that

P(x).P(y) = P(x + y) = P(y).P(x)

Given,

P(x) = …(1)

P(y) =

∴ P(x).P(y) =

⇒ P(x).P(y) =

We know that-

cos x cos y + sin x sin y = cos (x – y)

cos x sin y + sin x cos y = sin (x + y)

and cos x cos y – sin x sin y = cos (x + y)

⇒ P(x).P(y) =

In comparison with equation 1 we can say that:

…(2)

∴ P(x).P(y) = P(x + y)

Similarly, we can show for P(y).P(x):

P(y).P(x) =

By matrix multiplication, we have –

P(y).P(x) =

⇒ P(y).P(x) =

⇒ P(y).P(x) = …(3)

∴ From equation 2 and 3:

P(x).P(y) = P(y).P(x) = P(x + y) …ans