Find 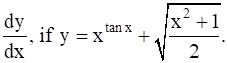
We have, ![]()
Putting xtanx = u and ![]()
u = xtanx
Taking log on both sides, we get
log u = tanx log x
Differentiating w.r.t x, we get
⇒ ![]()
⇒ ![]()
⇒ ![]() (i)
(i)
Now, ![]()
⇒ ![]()
Differentiating w.r.t x, we get
⇒ ![]()
⇒ ![]() (ii)
(ii)
Now, y = u + v
⇒ ![]()
On substituting the values of ![]() from (i) and (ii),we get
from (i) and (ii),we get
⇒ ![]()
⇒ ![]()
82