Find the equation of the curve through the point (1, 0) if the slope of the tangent to the curve any point (x, y) is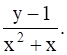

Question

Philoid · Accepted Answer

Slope of tangent is given as

Slope of tangent of a curve y = f(x) is given by

Integrate

Using partial fraction for

Equating numerator

⇒ A(x + 1) + Bx = 1

Put x = 0

⇒ A = 1

Put x = -1

⇒ B = -1

Hence

Hence equation (a) becomes

⇒ log(y – 1) = logx – log(x + 1) + c …(b)

Now it is given that the curve is passing through (1, 0)

Hence (1, 0) will satisfy the curve equation (b)

Putting values x = 1 and y = 0 in (b)

If we put y = 0 in (b) we get log (-1) which is not defined hence we must simplify further equation (b)

⇒ log (y – 1) – log x = – log (x + 1) + c

Using log a – log b = log

Using log a + log b = log ab

Take the constant c as log c so that we don’t have any undefined terms in our equation (Why only log c and not any other term because taking log c completely eliminates the log terms so we don’t have to worry about the undefined terms appearing in our equation)

Eliminating log

Now substitute x = 1 and y = 0

⇒ c = -2

Put back c = -2 in (c)

Hence the equation of curve is (y – 1)(x + 1) = -2x