Find the real values of θ for which the complex number 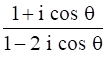is purely real.

Question

Philoid · Accepted Answer

Let us assume the given complex number as

Multiplying and dividing with 1+2icos

⇒

We know that i²=-1

⇒

For a complex number to be purely real, the imaginary part equals to zero.

⇒

⇒ 3cos=0 (∵ 1+4cos²θ≥1)

⇒ cos θ=0

⇒ , for nI

∴ The values of θ to get the complex number to be purely real is for nI.